Parallelogram Law

The parallelogram law from plane geometry

The sum of the squares of the lengths of two diagonals of a parallelogram is equal to the sum of the squares of all four sides.

Let O,P,S,Q be vertices of a parallelogram (see Fig 1 and Fig 2) Then
   |OS|² + |QP|² = |OP|² + |PS|² + |SQ|² + |QO|²
   |OS|² + |QP|² = |OP|² + |PS|² + |SQ|² + |QO|²
Because opposite sides of a parallelogram have equal lengths, these equations are equivalent to
($)    |OS|² + |QP|² = 2|OP|² + 2|OQ|²
($)    |OS|² + |QP|² = 2|OP|² + 2|OQ|²

Also let θ be the angle at O. So that a genuine parallelogram exists,
       0° < θ < 180°.
There are actually two situations (see Fig 3 and Fig 4):
     0° < θ ≤ 90° and 90° < θ < 180°.

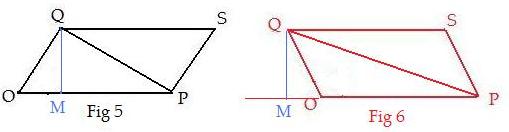
From vertex Q draw diagonal QP and drop a perpendicular down to line OP. Then pairs of right triangles are formed:
   triangle OMQ and PMQ
in both figures Fig 5 and Fig 6. By the Pythagorean theorem
  |OQ|² = |OM|² + |MQ|²,    |QP|² = |PM|² + |MQ|²
in both figures. Subtract the two equations to eliminate |MQ|² to get
(&)    |QP|² − |OQ|² = |PM|² − |OM|²
which is true for both parallelograms.

But now arguments for the black and red parallelograms are different.

In the black parallelogram, eliminate |PM|² by substitution |PM| = |OP| − |OM| whose square is
|PM|² = |OP|² − 2 |OP| |OM| + |OM|²
Inserting the right side of this equation for |PM|² in equation (&) produces
(@) |QP|² − = |OP|² + |OQ|² − 2 |OP| |OM|.

In the red parallelogram, eliminate |PM|² by substitution |PM| = |OP| + |OM| to get from (&)
(@) |QP|² − = |OP|² + |OQ|² + 2 |OP| |OM|.

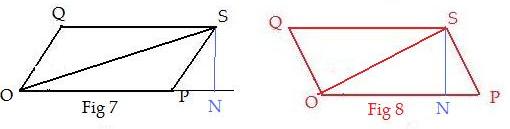
From vertex S draw diagonal OS and drop a perpendicular down to line OP. Then pairs of right triangles are formed:
   triangle ONS and PNS
in both figures Fig7 and Fig 8. By the Pythagorean theorem
  |PS|² = |PN|² + |NS|²,    |OS|² = |ON|² + |NS|²
in both figures. Subtract the two equations to eliminate |NS|² to get
   |OS|² − |PS|² = |ON|² − |PN|²
But |PS| = |OQ| so
(&&)    |OS|² − |OQ|² = |ON|² − |PN|²
which is true for both parallelograms.

But now arguments for the black and red parallelograms are different.

In the black parallelogram, eliminate |ON|² by substitution |ON| = |OP| + |PN| whose square is
|ON|² = |OP|² − 2 |OP| |PN| + |PN|²
Inserting the right side of this equation for |ON|² in equation (&&) produces
(%) |OS|² = OP² + |OQ|² + 2 |OP| |PN|.

In the red parallelogram, eliminate |ON|² by substitution |ON| = |OP| − |PN| to get from (&&)
(%) |OS|² = |OP|² + |OQ|² − 2 |OP| |ON|.

Actually all the black parallelograms are the same. So right triangle OQM and PSN are congruent. Hence |OM| = |PN|.
Similarly, OQM and PSN are congruent. Hence OM = PN. Equations (%) and (%) become
(@@) |OS|² = OP² + |OQ|² + 2 |OP| |OM|.
(@@) |OS|² = OP² + |OQ|² − 2 |OP| |OM|. .

Addition of equations (@) and (@@) and addition of equations (@) and (@@) produce the desired equations
|QP|² + |OS|² = 2|OP|² + 2|OQ|²
|QP|² + |OS|² = 2|OP|² + 2|OQ|²